The fact that the particle continues to oscillate implies that the system is underdamped. Underdamped motion is described by

$\begin{align}x(t) = A e^{- b t/2m}\cos \left(\sqrt{k^2/m^2 - b^2/(4 m^2)}t - \delta \right)\end{align}$
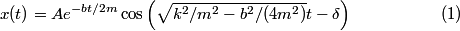(see Marion and Thornton pages 109 and 110).
Thus we see that the frequency is reduced compared to the frequency of the harmonic oscillations. So, the period must have increased. So, answer (A) is correct. The period of the underdamped motion is

$\setcounter{equation}{1}\begin{align}\frac{2 \pi}{\sqrt{k^2/m^2 - b^2/(4 m^2)}}\end{align}$
This makes it clear that answers (C), (D), and (E) are wrong.

Solution to 1996 Problem 8